A New Method for Prediction of Bearing Temperature Distribution
A schematic depiction of the algorithm of the new method is shown in (Fig. 1). The new method uses the results of friction-generated heat obtained by direct-coupled structural thermal analysis in the time domain as an input of transient thermal analysis. The input parameters of the direct-coupled analysis in the time domain are the coefficient of friction, radial force, and speed. As already noted, the result of the direct-coupled analysis is the value of the generated heat flux at nominal operating conditions; in the nominal operating conditions the obtained heat flux is constant over time.
Figure 1 Schematic depiction of the algorithm for the prediction of bearing temperature distribution.
The determined value of the contact heat flux can be averaged and can be used as a boundary condition in the transient thermal analysis. In this way it is possible to determine the temperature field of bearings at any point in time. The advantage of the proposed procedures is the fact that with the use of moderate computer resources, and for a relatively short time, it is possible to determine the temperature of the bearing. Use of only direct-coupled analysis is not rational because it would require vast computational resources to obtain the temperature distribution in reasonable time. Unlike other authors who mathematically determined the contact heat flux and then used calculated flux in thermal simulations, for the first the time generated heat flux due to friction in the bearings is determined by simulation.
For a proposed new method, a case study was defined. The goal of the case study was to obtain temperature distribution of bearing 6310 made from 100Cr6 steel, subjected to radial load of 4,600 N at a rotation speed of 1,140 rpm. Numerical analysis was performed in ANSYS Workbench. Model loads and boundary conditions were defined using joints in order to take into account the rotation of the shaft and the bearing pressure applied to the shaft (Fig. 2). Heat transfer from the model of the environment is defined from all the surfaces with a heat transfer coefficient of 15 W/m2K. It was necessary to define the convection over command interface, as ANSYS Workbench currently does not have a graphical user interface for direct structural-thermal coupling. The thermal material properties of bearing steel 100Cr6 are given (Table 1). Properties of the material during the analysis were regarded as constant since the simulation time was short (2 seconds) as the goal of this analysis was to obtain contact flux only and the anticipated temperatures were below 100°C.
Figure 2 Loads and boundary conditions of a structural-thermal direct coupled analysis.
In order to perform a direct coupling of the thermal and structural field, was used in the final element SOLID 226, ANSYS (Ref. 8) to generate a finite element mesh. The discrete model is composed of 59,012 nodes that form 15,989 elements. The contacts between the raceways and rolling elements were defined as the frictional contact, with the friction coefficient value of 0.08. The contacts were treated as symmetrical, with the use of the extended Lagrange formulation. Stiffness of contacts was automatically updated at every iteration of numerical solution. Software has been assigned to the account for the heat generated by friction through the command interface. Figure 3 shows the distribution of heat flux between the rolling bodies and the inner bearing ring. It is obvious that the heat flux is generated only in contacts were the rolling bodies are in contact with the rolling surface as a consequence of the action of radial load.
Figure 3 Boundary conditions of thermal analysis in time domain.
The discrete model is transferred to the thermal analysis in the time domain. The obtained contact flux from that previously given was averaged and used as a load in thermal analysis; model and the boundary conditions are shown (Fig. 4).
Figure 4 Boundary conditions of thermal analysis in time domain.
The result of transient thermal analysis is a temperature distribution of the bearing. Results obtained by simulation were compared with the results obtained experimentally for an experimental setup that corresponds to the above defined case study (Fig. 5). It is clear from Figure 5 that there is a reasonable agreement between the results obtained by a proposed new method and the experimental ones.
Figure 5 Comparison of numerically and experimentally obtained results.
Conclusion
This paper presents a new method for determination of the temperature distribution of the transmission bearing; the method consists of two analyses by finite element method performed in succession. The first, directly coupled structural thermal analysis, gives a heat flux generated due to friction at the contact of rolling bodies and the raceways. The resulting heat flux is then averaged on the raceway surface and used as a boundary condition in transient thermal analysis that, as a result, reveals the temperature distribution of the bearing. The performed case study presented here showed that the new method predicts bearing temperature distribution reasonably well, as there is a good agreement between the results obtained by FEM and experimentation. The new method presented in the paper is reasonably straightforward to perform, computationally efficient, and can be expanded to account for temperature-dependent thermal properties. Further research should be directed towards validation of the method for the more realistic conditions, such as existence of radial clearance, friction generation in the contact of the rolling elements with a cage, as well as other influences that contribute to friction heat generation. 
References
- Vats, P., B.C. Sharma and S. Sharma. “Heat Transfer through Journal Bearing: A Case Study,” International Journal of Research in Engineering and Technology ,eISSN: 2319-1163 | pISSN: 2321-7308.
- Kushwaha A.S., A.B. Wankhade, D.E. Mahajan and D.K. Thakur. “Analysis of the Ball Bearing considering the Thermal (Temperature) and Friction Effects,” International Journal of Engineering Research and Applications (IJERA) ISSN: 22489622 National Conference on Emerging Trends in Engineering & Technology (VNCET30 Mar’12), pp. 115–120.
- Ulysee, P. and M.M. Khonsari. “Thermal Response of Rolling Components under Mixed Boundary Conditions: An Analytical Approach,” ASME Journal of Heat Transfer, 1993, 115, 857–865.
- Hazlett, T.L. and M.M. Khonsari. “Finite Element Model of Journal Bearing Undergoing Rapid Thermally Induced Seizure,” Tribology International, 1992a, 25, 177–82.
- Hazlett, T.L. “Thermo-Elastic Behavior of Journal Bearing Undergoing Seizure — a Finite Element Study,” M.S. Thesis, University of Pittsburgh, Pittsburgh, Pennsylvania, 1990.
- Wang, H., T.F. Conry and C. Cusano. “Effects of Cone/Axle Rubbing Due to Roller Bearing Seizure on the Thermomechanical Behavior of a Railroad Axle,” ASME Journal of Tribology, 1996, Vol. 118, pp. 311–319.
- Wang, H. “Axle Burn-off and Stack-up Force Analyses of a Railroad Roller Bearing using the Finite Element Method,” Ph.D. Thesis, Department of Mechanical and Industrial Engineering, University of Illinois at Urbana Champaign, 1996, p 35, pp. 140–147. 75 1.
- ANSYS theory manual.
 Aleksandar Miltenović
Aleksandar Miltenović is assistant professor at Faculty of Mechanical Engineering, University of Niš. He received his PhD at Ruhr University of Bochum in 2011. His current research interests include power transmission (especially of worm gears) and product development.
 Milan Banić
Milan Banić is assistant professor at Faculty of Mechanical Engineering, University of Niš. He received his PhD at the University of Niš in 2015. His current research interests include tribology, power transmission and product development.
 Žarko Mišković
Žarko Mišković is assistant professor at Faculty of Mechanical Engineering, University of Belgrade. He received PhD at the University of Niš in 2017. His current research interests include bearings and product development.
 Radivoje Mitrović
Radivoje Mitrović is full professor and Dean at Faculty of Mechanical Engineering, University of Belgrade. He received his PhD at the University of Belgrade in 1992. His current research interests include bearings and technical design.
 Vojislav Miltenović
Vojislav Miltenović is full professor and works as Deputy Director at the Innovation Centre of University of Niš. He received his PhD at the University of Niš in 1982. His current research interests include technical design, product development and innovation.
This paper was first presented at the International VDI Conference on Gears 2017, Garching/Munich [VDI-Berichte 2294, 2017, VDI Verlag GmbH] and is reprinted here with VDI approval.




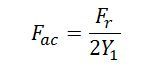







 Aleksandar Miltenović is assistant professor at Faculty of Mechanical Engineering, University of Niš. He received his PhD at Ruhr University of Bochum in 2011. His current research interests include power transmission (especially of worm gears) and product development.
Aleksandar Miltenović is assistant professor at Faculty of Mechanical Engineering, University of Niš. He received his PhD at Ruhr University of Bochum in 2011. His current research interests include power transmission (especially of worm gears) and product development. Milan Banić is assistant professor at Faculty of Mechanical Engineering, University of Niš. He received his PhD at the University of Niš in 2015. His current research interests include tribology, power transmission and product development.
Milan Banić is assistant professor at Faculty of Mechanical Engineering, University of Niš. He received his PhD at the University of Niš in 2015. His current research interests include tribology, power transmission and product development. Žarko Mišković is assistant professor at Faculty of Mechanical Engineering, University of Belgrade. He received PhD at the University of Niš in 2017. His current research interests include bearings and product development.
Žarko Mišković is assistant professor at Faculty of Mechanical Engineering, University of Belgrade. He received PhD at the University of Niš in 2017. His current research interests include bearings and product development. Radivoje Mitrović is full professor and Dean at Faculty of Mechanical Engineering, University of Belgrade. He received his PhD at the University of Belgrade in 1992. His current research interests include bearings and technical design.
Radivoje Mitrović is full professor and Dean at Faculty of Mechanical Engineering, University of Belgrade. He received his PhD at the University of Belgrade in 1992. His current research interests include bearings and technical design. Vojislav Miltenović is full professor and works as Deputy Director at the Innovation Centre of University of Niš. He received his PhD at the University of Niš in 1982. His current research interests include technical design, product development and innovation.
Vojislav Miltenović is full professor and works as Deputy Director at the Innovation Centre of University of Niš. He received his PhD at the University of Niš in 1982. His current research interests include technical design, product development and innovation.